21. h&k are parallel as are j&l. How many pairs of lines? 2 (not 4).
22. Since each week has 7 days, every multiple of 7 will also be a Sunday. 49 days would be a Sunday, so 50 days is the next day, Monday.
23. Reading the graph, the percentage (represented by the dotted line) of antifreeze needed at temperature = -20 is 40%.
24. Convert the fractions to decimals and Plug In!. 1/4 = 0.25 = 0.N5. 1/2 = 0.5 = 0.M
Therefore, N = 2 and M = 5. N + M = 2 + 5 = 7
25. Backsolve.
The circumference of circle A = dπ = 2(100)π = 200π. We're looking for a circumference for circle B that is half of 200π --> 100π
c) r = 20, d = 40. circumference = 40π --> Too Small, move down (larger radius).
d) r = 50, d = 100. circumference = 100π --> bingo.
26. Plug In! If k = 2,
27. The absolute value of g(x) means that the y values that are positive stay positive, but the y values that are negative are made positive. Since f(x) = 2 for every value of x, we're looking for how many points have an x coordinate such that |g(x)| > 2. Let's see:
Point a has a y value of 2, no.
Point b has a y value of about -2.5, |-2.5| = 2.5, yes.
Point c has a y value of about 2.5, yes
Point d has a y value of about -0.5, |-0.5| = 0.5, no.
Only point b and c work.
28. For (x - 2) and (x + 2) to both be factors, (x - 2)*(x + 2) must be a factor as well.
Factoring out 100 from the polynomial equation, we can isolate the variables:
From here, we can Backsolve. What value for k would allow us to get some multiple of x2 - 4?
The answer is k = -400.
Or algebraically:
Grid-Ins:
29. Adding a hundredths digit might make this easier (than it already is) to visualize. What number is between 0.30 and 0.40? 0.35.
30. Simply plug in the different x values to the function c = 1200 + 2x
x = 5, c = 1200 + 25 = 1232
x = 1, c = 1200 + 21 = 1202
1232 - 1202 = 30
31. Because the two triangles, ABE and ACD are similar, the ratio of AB to AC is the same as the ratio of AE to AD.
AB/AC = 2/3 so AE/AD is in that same ratio.
2/3 = AE / 12, cross multiply --> 3*AE = 24, AE = 8
32. 
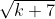must be a perfect square greater than 5.
Since k must also be between 0 and 60 only 36, 49, and 64 work.
k = 36 - 7 = 29 or 42 - 7 = 42 or 64 - 7 = 57
All three work.
33. l = 3w. The perimeter is 2l + 2w = 2(3w) + 2w = 8w
8w = 840, w = 105. This is not the answer! RTFQ!
3w = l = (105)*3 = 315
34. 3y = (4)*(5) = 20, y = 20/3
To more easily to the math, convert x to a fraction as well.
y - x = 20/3 - 15/3 = 5/3
35. The question forces you to find the value for the region marked with the x on this Venn diagram. That is the segment of students who do all three activities (sports, drama, and music). All of the 250 students are accounted for, except the x region.
58 + 21 + 26 + 35 + 50 + 40 = 230. This means that x = 20. Now that we know this, we can figure out how many students who participate in sports also participate in drama. Be careful here. Just because the student does music as well does not exclude him/her from being counted as a student who does sports and drama.
21(sports and drama) + 20(all 3) = 41.
21(sports and drama) + 20(all 3) = 41.
36. AVERAGE TABLE!
# x AVG = SUM
20 x 9.5 = 190
10 x 8.3 = 83
+ 30 = 273
273 / 30 = 9.1
37. If there are 2 slots for letters and 1 slot for a number, how many possible choices are there for each of the 3 slots?
26 for the 1st, 26 for the 2nd (since we know that the letters are not necessarily different), and 10 for the 3rd (0 through 9). To get the total number of outcomes, multiply those numbers.
26 * 26 * 10 = 6,760
38. Plug In! A good way to think about this is to think of cubes with sides of 1 and 4. Cubes with sides 1 and 4 have volumes of 1 and 64, respectively. Now, each cube's volume is 10 times bigger, but the relationship is the same. Remember analogies? Ok, you probably don't, but 1 : 64 as 10 : 640.
Posts
